Editor’s note: We’re revisiting this series on navigating by the sun, moon, stars and planets in the age of GPS because celestial nav is not only a viable backup to satellite navigation, but it is also a skill that ocean voyagers should have in their toolkit. During the next few issues, we’ll cover all the basic knowledge you’ll need to get up to speed on this elegant and rewarding technique for finding your way at sea.
A thumbnail sketch
Imagine yourself as a little stick figure of a human standing somewhere on the Earth’s surface. That’s your position. You’re not exactly sure where it is in terms of latitude and longitude, but you have a good idea. Call that your dead reckoning (DR) position.
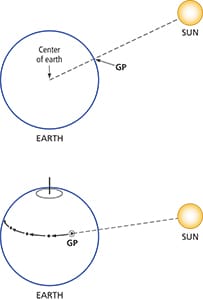
Now also imagine that the sun’s light is shining directly down onto the Earth’s surface, and where it hits the surface there is a little X marking the spot, as if it were under a magnifying glass. Call that the sun’s geographic position (GP).
Now we have the image of the Earth in mind with two things on its surface: your little stick figure marking your DR position, and an X marking the GP of the sun. The essence of celestial navigation, as we practice it today, is in defining, measuring and plotting the distance between these two spots. Clearly, if both spots can be marked on the Earth’s surface, it would be possible to measure the distance between them. If we knew how far we were from the sun’s GP at a given moment, then we could draw a circle on the Earth with a radius equal to that distance, and we could say we were somewhere on that circle of position.
 |
|
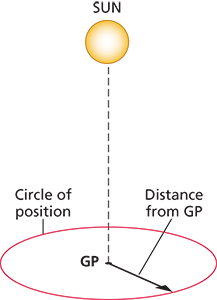
At a given distance from the GP, we have a circle of equal altitude. |
Well, it so happens that with a sextant it is very easy to measure the distance between our two spots. The distance between your own position and the sun’s GP is directly related to the altitude of the sun as measured by the sextant. The higher the altitude, the closer you are to the GP. If the sun were directly overhead, you would be right at the GP or very close to it, and our circle of position would be quite small. If the sun is low down on the horizon, you may be thousands of miles from the GP and the circle of position would be similarly sized. But either way, your own position would be somewhere on one of those circles. You can carry this a step further by using one of the basic rules of thumb for celestial navigation: Subtract the measured sextant altitude from 90 degrees and multiply the result by 60, and that is your distance from the GP in nautical miles. For instance, if the sextant altitude is 72 degrees, as it might often be at midday in lower latitudes, you would be 1,080 miles from the GP.
Now let’s switch to evening twilight as you begin to see the stars just after sunset. Imagine arrows of light coming directly from two of those stars toward the center of the Earth. Where the arrows hit the Earth’s surface, we have two more GPs. And if we measured the altitude of each star with our sextant, we would have two circles of position on which we were located. We know from basic navigation theory that if we are located on two different lines or circles of position, we must be located at the intersection of those lines that is closest to our DR position.
Thus, we have determined our position on the Earth’s surface. There are a few additional items that beg for inclusion in this thumbnail sketch, however. First, we are entirely omitting any mention of the celestial triangle, which allows us so simply to equate measured altitude with distance to the GP of a celestial object. Second, there are several corrections that need to be made to any sextant reading, without which there could be no semblance of accuracy. And third, it would be virtually impossible to plot a circle of position thousands of miles across, let alone two or three of them, in such a way as to allow a navigator to determine their position with any accuracy.
 |
|
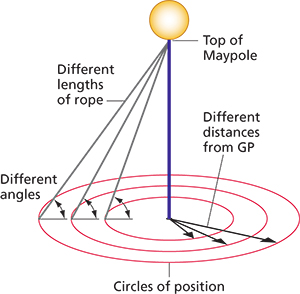
Different lengths of rope from the top of a Maypole would produce different angles, which would correspond to distance from the GP. |
For this presentation, we’ll gladly skip the celestial triangle. And the sextant corrections? Mere details. The only problem thus far is in finding a way to plot our circles. Let’s look at that problem in a little more detail.
The primary purpose of the collection of data in the Nautical Almanac is to provide us with the precise location of the GP of the observed body at the instant we took our sextant sight. And, from our ship’s dead reckoning plot, we are able to provide a specific latitude and longitude for what we believe our position to be at the time of the sight. We do, therefore, know the location of each of the points on our DR and the GP on the Earth’s surface.
Since these points may be thousands of miles away from each other, we would find it difficult to plot them both on any kind of usable chart. In practice, our plotting technique will be to omit the point of the GP and only to plot our position on a chart that includes our own little corner of the ocean. But we’re not sure of our own position. It’s only a DR position.
So, the navigator plots both their own DR position and a nearby, arbitrarily chosen position, called an assumed position (AP). Now we are into what is called sight reduction. The navigator has a set of tables that have pre-calculated exactly what the altitude of the observed body should have been at the moment of observation had the sight been taken from the assumed position. This calculated altitude is typically different from the actual observed altitude. The difference between the two angles might be as much as a half-degree or even more. What is this difference? It is the difference in distance between the AP and the GP and the distance from your real position to the GP.
 |
 |
|
|
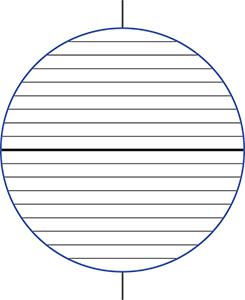
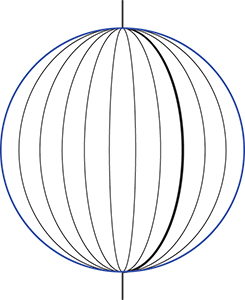
Left, latitude on Earth is set up in parallels from the equator to 90° N and to 90° S. The distance between degrees of latitude is fixed. Longitude, right, has meridians that converge at the poles and are widest apart at the equator. Distance between meridians is not fixed. |
||
This difference tells you that at your real position, you are so many miles closer or further from the GP than the assumed position. And since we have plotted the assumed position on our chart, we can begin to visualize our own position on the chart as being so many miles from the assumed position in a direction relative to the direction to the GP (this direction is also provided by the sight reduction tables).
What all this boils down to is that we never plot the entire circle of position around the GP. The navigator only plots a tiny portion of the circle in the vicinity of the AP. The portion of the circle that actually gets plotted is, in effect, a straight line, almost a tangent of the much larger circle. Thus, the navigator comes up with a straight line, a Line Of Position (LOP) that they are able to derive from their celestial observation. This LOP will pass very close, hopefully, to their DR position. And, with a bit of luck and skill, they may come up with two or three other LOPs at the same time (from other stars, planets or the moon, perhaps).
That’s the basic idea of celestial navigation. From this point forward, we’ll look at specific elements of this process. We’ll go into detail on each practical step and provide you with the knowledge to complete them.
Before we do that, however, let’s review some navigation fundamentals such as latitude and longitude and nautical charts.
 |
|
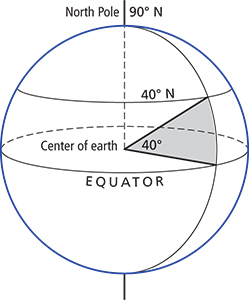
Latitude is an angle at the equator. |
Latitude and longitude
How do we determine positions on the Earth? We use a grid system that allows us to find any position using two sets of numbers. The grid system is called, of course, latitude and longitude. There are 60 minutes in each degree of latitude and longitude.
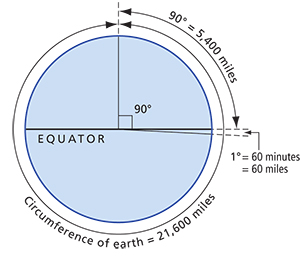
Lines of latitude measure north and south. Latitude can be thought of as an angle from the equator to the center of the Earth and then back out to the surface. Thus the North Pole is at 90° N while an angle for 40° would lead to the line at 40° N. This gives latitude lines some useful characteristics: Each line of latitude is parallel to every other line (these lines are called “parallels”); even better, there is the same distance between each pair of parallels of latitude. In practice, each minute of latitude equals one nautical mile. The easiest way to measure distance is from the latitude scale at the side of the chart.
Meridians of longitude differ from latitude in that they are not parallel. They resemble slices of an orange as they converge at the poles. Thus, the distance between each degree of longitude varies from about 60 miles at the equator to nothing at the poles. Minutes of longitude cannot be used to measure distance. This varying amount of distance between lines of longitude — the amount of difference that is based on latitude — means that attempts to put the curved surface of the Earth on a flat chart leads to some distortion.
 |
||
|
Left, declination works just like latitude (0° to 90° N and 90° S). Right, Greenwich Hour Angle (GHA) is measured from 0°, starting at the Greenwich Meridian, through 360°. |
Charts
There are many types of map projections: Mercator, Polyconic, Lambert conformal conic, Gnomonic and even something called Loximuthal.
The type of projection we use most often in marine navigation is Mercator. And like other map/chart projections, Mercator charts do involve distortion. However, this distortion is insignificant on the Mercator projection charts that navigators use for coastal or near-coastal work. The Mercator projection is so useful that we will use it when constructing our own charts for celestial work.
Pilot charts provide a tremendous amount of meteorological and oceano-graphic information about the ocean that they cover. These are Mercator projections but they are too cluttered with information to be of much use for plotting. They are best used for planning and as a resource while underway.
 |
|
The relationship between latitude and distance: one degree of latitude is equal to 60 miles. |
Gnomonic projections are used to determine courses and distances for great circle routes and as a result are not used frequently by most navigators.
Polyconic charts are used in the Great Lakes. They do not offer any advantage over a Mercator projection and their use is the result of surveying and chart-making techniques used on the Great Lakes.
Almost all charts used for navigation are Mercator projections. These have one tremendous advantage over other types of projections: Distance and direction are accurately shown and can be taken directly from the chart.
This is of great advantage to the navigator. Distance can simply be determined from the latitude scale because one minute of latitude is always equal to one mile. Direction can be taken from the compass rose and accurately applied to courses or bearings anywhere on the chart.
In simple terms, a Mercator projection has the meridians of longitude straightened so that they, as well as the lines of latitude, are parallel. This creates distortion. On small-scale charts, like those covering the entire North Atlantic, the latitude scale changes significantly as you move north or south, so try to use the scale appropriate to your latitude.
 |
 |
|
|
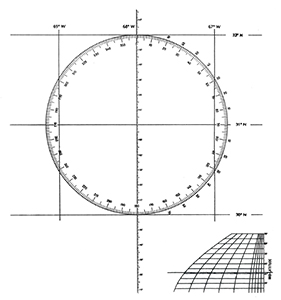
The familiar nautical chart is based on a Mercator projection, left. This gives us parallel lines of longitude (with a small bit of distortion for a chart of an area of this size). Another Mercator chart is a Universal Plotting Sheet, right. |
||
Universal Plotting Sheets
Universal Plotting Sheets allow a navigator to build their own custom Mercator chart for virtually any position in the world. Lines of latitude are already printed on the sheet. These are of great value for any offshore work where large-scale charts don’t exist. It is also possible to buy Position Plotting Sheets, which have a larger scale than Universal Plotting Sheets and allow more precise navigation. They come by latitude and have the lines of longitude preprinted. The sheets are much larger than the Universal Plotting Sheets and are also much more expensive.
A celestial navigator can set up a Universal Plotting Sheet based on their latitude and then use that chart for several days of celestial navigation plotting. This makes the Universal Plotting Sheet an essential tool for the navigator.